Logic Gates Verilog Code

Logic gates are the building block of digital circuit and system. We can make any digital circuit using logic gates. The are three basic logic gates AND, OR and NOT gate, two universal gate NAND and NOR and two other logic gates Ex-OR and EX-NOR. In this post, how to write Verilog code for logic gates is discussed.

There are three Verilog codes for each logic gate, you can use any one code.

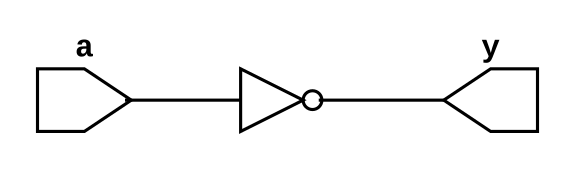
NOT Gate

NOT gate has one input and one output and both are complement of each other. If input is 1 output is 0 and vice versa. The truth table of NOT gate is given below and we can write bolean expression of NOT gate as $$y = \overline a \text{ or } y = a'$$ $$\text{Where a is input and y is output}$$

NOT Gate Truth Table

Input a	Output y
0	1
1	0

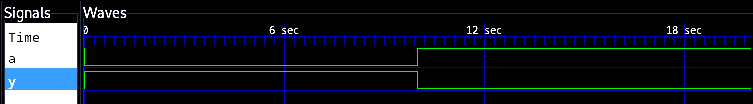
NOT Gate Verilog Code

//NOT gate using Structural modeling
module not_gate_s(a,y);
input a;
output y;

not(y,a);
                
endmodule

//NOT gate using data flow modeling
module not_gate_d(a,y);
input a;
output y;

assign y = ~a;
                
endmodule

//NOT gate using behavioural modeling
module not_gate_b(a,y);
input a;
output reg y;

always @ (a)
y = ~a;
                
endmodule

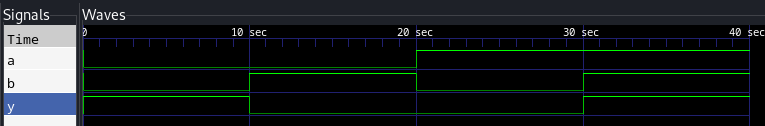
NOT Gate Testbench

module not_gate_tb;
reg a;
wire y;

not_gate_s uut(a,y);

initial begin
a = 0;
#10
b = 1;
#10
$finish();
end
                
endmodule

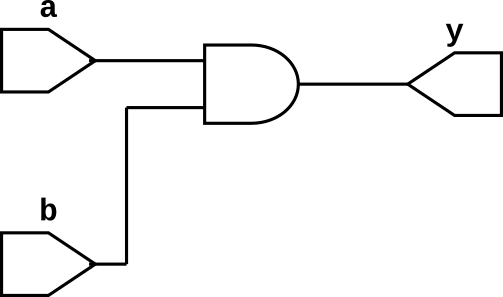
AND Gate

AND gate has many inputs (it can be two or more than two inputs) and one output. Output of the AND gate is 1 if and only if all of the inputs are 1. The truth table of 2-input AND gate is given below and we can write boolean expression for AND gate as follows $$y = ab \text{ or } y = a.b$$

AND Gate Verilog Code

//AND gate using Structural modeling
module and_gate_s(a,b,y);
input a,b;
output y;

and(y,a,b);
                
endmodule

//AND gate using data flow modeling
module and_gate_d(a,b,y);
input a,b;
output y;

assign y = a & b;
                
endmodule

//AND gate using behavioural modeling
module AND_gate_b(a,b,y);
input a,b;
output y;

always @ (a,b)
y = a & b;
                
endmodule

AND Gate Testbench

module and_gate_tb;
reg a,b;
wire y;

and_gate_s uut(a,b,y);

initial begin
a = 0; b = 0;
#10
b = 0; b = 1;
#10
a = 1; b = 0;
#10
b = 1; b = 1;
#10
$finish();
end
                
endmodule

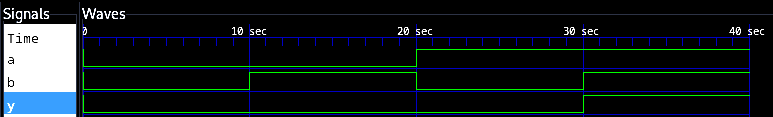
OR Gate

OR gate has many inputs (it can be two or more than two inputs) and one output. Output of the OR gate is 1 if one or more than one inputs are 1. The truth table of 2-input OR gate is given below and we can write boolean expression for OR gate as follows $$y = a + b$$

OR Gate Verilog Code

//OR gate using Structural modeling
module or_gate_s(a,b,y);
input a,b;
output y;

or(y,a,b);
                
endmodule

//OR gate using data flow modeling
module or_gate_d(a,b,y);
input a,b;
output y;

assign y = a | b;
                
endmodule

//Not gate using behavioural modeling
module or_gate_b(a,b,y);
input a;
output y;

always @ (a,b)
y = a | b;
                
endmodule

OR Gate Testbench

module or_gate_tb;
reg a,b;
wire y;

or_gate_s uut(a,b,y);

initial begin
a = 0; b = 0;
#10
b = 0; b = 1;
#10
a = 1; b = 0;
#10
b = 1; b = 1;
#10
$finish();
end
                
endmodule

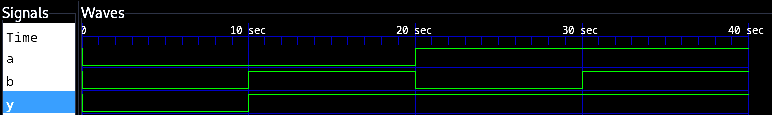
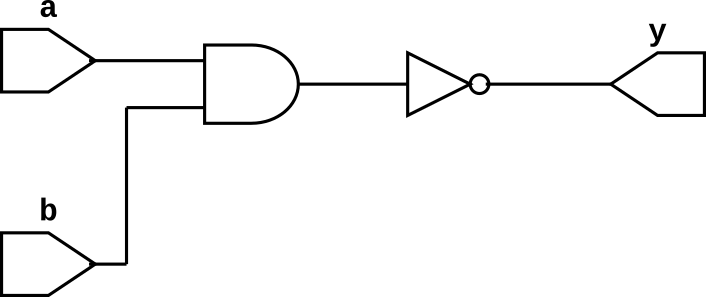
NAND Gate

NAND gate has many inputs (it can be two or more than two inputs) and one output. It is AND gate followed by NOT gate and output of the NAND gate is 0 if all inputs are 1 else it is 1. The truth table of 2-input NAND gate is given below and we can write boolean expression for OR gate as follows $$y = \overline{ab} \text{ or } \overline{a.b}$$

NAND Gate Verilog Code

//NAND gate using Structural modeling
module nand_gate_s(a,b,y);
input a,b;
output y;

nand(y,a,b);
                
endmodule

//NAND gate using data flow modeling
module nand_gate_d(a,b,y);
input a,b;
output y;

assign y = ~(a & b);
                
endmodule

//NAND gate using behavioural modeling
module nand_gate_b(a,b,y);
input a;
output reg y;

always @ (a,b)
y = ~(a & b);
                
endmodule

NAND Gate Testbench

module nand_gate_tb;
reg a,b;
wire y;

nand_gate_s uut(a,b,y);

initial begin
a = 0; b = 0;
#10
b = 0; b = 1;
#10
a = 1; b = 0;
#10
b = 1; b = 1;
#10
$finish();
end
                
endmodule

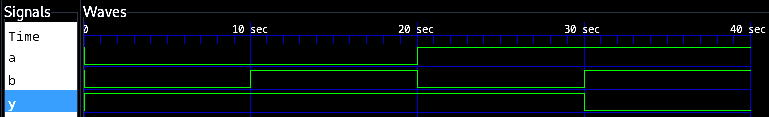
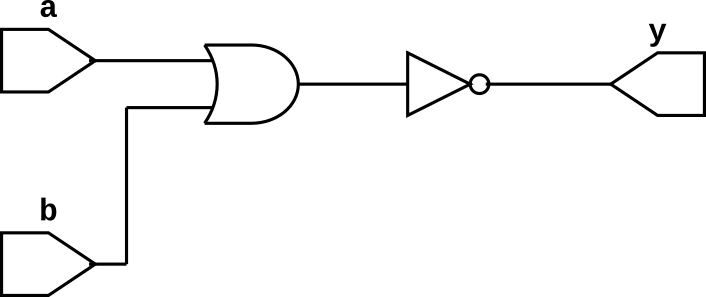
NOR Gate

NOR gate has many inputs (it can be two or more than two inputs) and one output. It is NOR gate followed by NOT gate and output of the NOR gate is 1 if all inputs are 0 else it is 1. The truth table of 2-input NAND gate is given below and we can write boolean expression for OR gate as follows $$y = \overline{a+b}$$

NOR Gate Verilog Code

//NOR gate using Structural modeling
module nor_gate_s(a,b,y);
input a,b;
output y;

nor(y,a,b);
                
endmodule

//NOR gate using data flow modeling
module nor_gate_d(a,b,y);
input a,b;
output y;

assign y = ~(a | b);
                
endmodule

//NOR gate using behavioural modeling
module nor_gate_b(a,b,y);
input a;
output reg y;

always @ (a,b)
y = ~(a | b);
                
endmodule

NOR Gate Testbench

module nor_gate_tb;
reg a,b;
wire y;

nor_gate_s uut(a,b,y);

initial begin
a = 0; b = 0;
#10
b = 0; b = 1;
#10
a = 1; b = 0;
#10
b = 1; b = 1;
#10
$finish();
end
                
endmodule

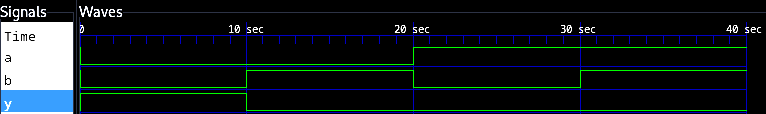
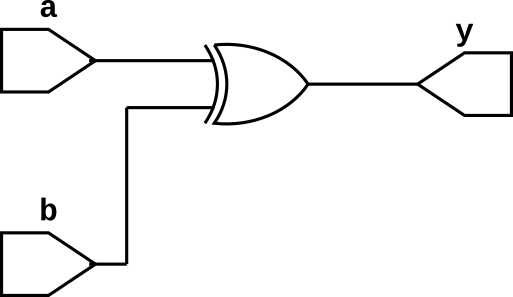
EX-OR Gate

EX-OR gate has many inputs (it can be two or more than two inputs) and one output. The output of EX-OR gate is 1 if odd number of inputs are 1 else it is 0. The truth table of 2-input EX-OR gate is given below and we can write boolean expression for OR gate as follows $$y = a\oplus b$$

EX-OR Gate Verilog Code

//EX-OR gate using Structural modeling
module xor_gate_s(a,b,y);
input a,b;
output y;

xor(y,a,b);
                
endmodule

//EX-OR gate using data flow modeling
module xor_gate_d(a,b,y);
input a,b;
output y;

assign y = a ^ b;
                
endmodule

//EX-OR gate using behavioural modeling
module xor_gate_b(a,b,y);
input a;
output reg y;

always @ (a,b)
y = a ^ b;
                
endmodule

EX-OR Gate Testbench

module xor_gate_tb;
reg a,b;
wire y;

xor_gate_s uut(a,b,y);

initial begin
a = 0; b = 0;
#10
b = 0; b = 1;
#10
a = 1; b = 0;
#10
b = 1; b = 1;
#10
$finish();
end
                
endmodule

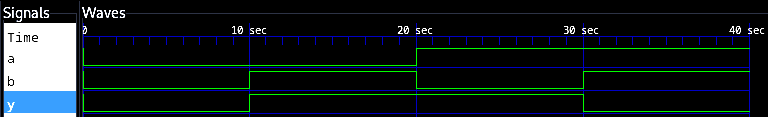
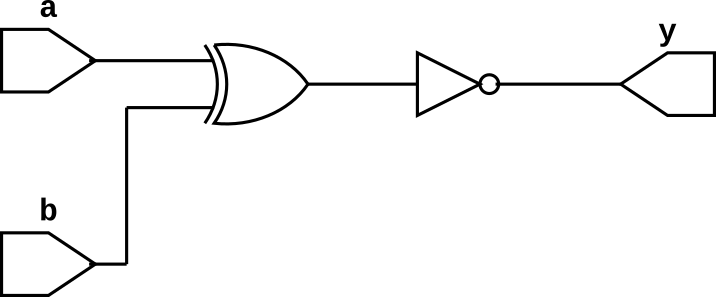
EX-NOR Gate

EX-NOR gate has many inputs (it can be two or more than two inputs) and one output. The output of EX-NOR gate is 1 if even number of inputs are 1 else it is 0. The truth table of 2-input EX-NOR gate is given below and we can write boolean expression for OR gate as follows $$y = \overline{a\oplus b}$$

EX-NOR Gate Verilog Code

//EX-NOR gate using Structural modeling
module xnor_gate_s(a,b,y);
input a,b;
output y;

xnor(y,a,b);
                
endmodule

//EX-NOR gate using data flow modeling
module xnor_gate_d(a,b,y);
input a,b;
output y;

assign y = ~(a ^ b);
                
endmodule

//EX-NOR gate using behavioural modeling
module xor_gate_b(a,b,y);
input a;
output reg y;

always @ (a,b)
y = ~(a ^ b);
                
endmodule

EX-OR Gate Testbench

module xnor_gate_tb;
reg a,b;
wire y;

xnor_gate_s uut(a,b,y);

initial begin
a = 0; b = 0;
#10
b = 0; b = 1;
#10
a = 1; b = 0;
#10
b = 1; b = 1;
#10
$finish();
end
                
endmodule