Universal Logic Gates NAND and NOR

With the help of NAND and NOR gate, we can implement any logical realisation that's why these gates are known as universal gates.

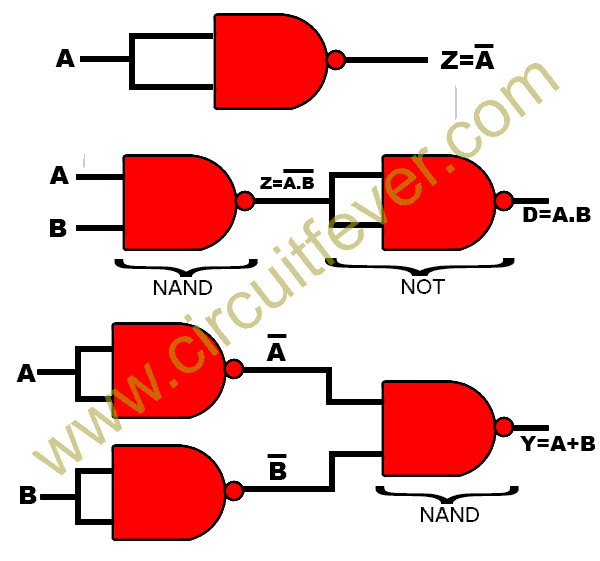
• NAND gate

AND gate followed by a NOT gate is known as NAND gate. NAND gate may have two or more inputs and only one output. The output of the NAND gate is '0' if all the inputs are '1'. For other inputs output is '0'.

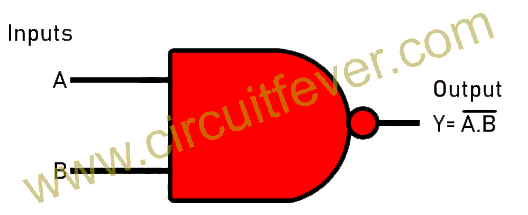
The output of the two inputs NAND gate is written by

$$Y= \overline {A.B} $$

The truth table of a two-input NAND gate is given by

Input A	Input B	Output Y
0	0	1
0	1	1
1	0	1
1	1	0

With the help of this gate, we can implement any logical expression.

Three basic gates are AND OR and NOT gate and these gates can be realised using NAND gate as given below.

NOT gate

If both the inputs of the NAND gate are same the output of the NAND gate is equal to the NOT of input.

i.e LET X and Y are the inputs of the NAND gate and X=Y=A then output Z becomes

$$Z=\overline {X.Y} \text { but } X=Y=A$$

$$Z=\overline{A.A}$$

$$Z=\bar A$$

Which is NOT operation.

AND Gate

If the output of NAND is connected by NOT gate (Which is implemented by a NAND gate) output becomes AND operation. Let X and Y are the inputs of the NAND gate also, let the input of NOT gate is C and output is D.

The output of the NAND gate becomes

$$Z=\overline {X.Y}$$

Z is connected to C. So, the output of NOT gate becomes

$$D=\overline{\overline{X.Y}}=X.Y$$

Which is AND operation

OR gate

Or gate is can be implemented by using three NAND gate.

Let A and B are the inputs of the NAND gate and Y is output. Then the output becomes

$$Y=\overline{A.B}$$

Now according to De Morgan's Theorem

$$\overline{A.B}=\overline A+\overline B$$

If inputs are

$$\overline A \text{ and } \overline{B}$$

Then outputs become

$$Y=\overline{\bar A.\bar B}=\overline{\bar A+\bar B}=A+B$$

Which is OR operation

With the help of NAND and NOR gate, we can implement any logical realisation that's why these gates are known as universal gates.

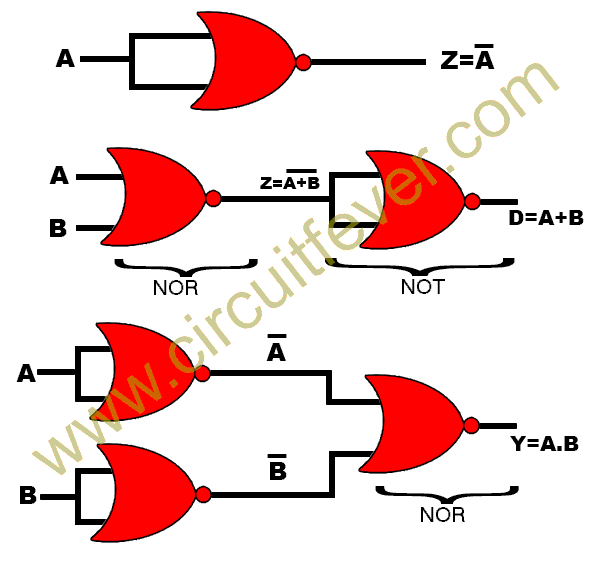
• NOR gate

OR gate followed by a NOT gate is known as NOR gate. NOR gate may have two or more inputs and only one output. The output of the NOR gate is '1' if all the inputs are '0'. For other inputs output is '0'.

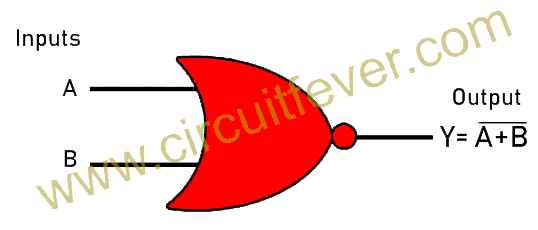
The output of the two inputs NOR gate is written by

$$Y=\overline{A+B}$$

The truth table of a two-input NOR gate is given by

Input A	Input B	Output Y
0	0	1
0	1	0
1	0	0
1	1	0

With the help of this gate, we can implement any logical expression.

Three basic gates are AND OR and NOT gate and these gates can be realised using NAND gate as given below.

NOT gate

If both the inputs of the NOR gate are the same the output of NOR gate is equal to the NOT operation.

i.e LET X and Y are the inputs of the NOR gate and X=Y=A then output Z becomes

$$Z=\overline {X+Y} \text { but } X=Y=A$$

$$Z=\overline{A+A}$$

$$Z=\bar A$$

Which is NOT operation.

OR Gate

If the output of NOR is connected by NOT gate (Which is implemented by a NOR gate) output becomes AND operation. Let X and Y are the inputs of the NOR gate also, let the input of NOT gate is C and output is D.

The output of the NOR gate becomes

$$Z=\overline {X+Y}$$

Z is connected to C. So, the output of NOT gate becomes

$$D=\overline{\overline{X+Y}}=X+Y$$

Which is AND operation

AND gate

AND gate can be implemented by using three NOR gate.

Let A and B are the inputs of the NOR gate and Y is output. Then the output becomes

$$Y=\overline{A+B}$$

Now according to De Morgan's Theorem

$$\overline{A+B}=\overline A . \overline B$$

If inputs are

$$\overline A \text{ and } \overline{B}$$

Then outputs become

$$Y=\overline{\bar A+\bar B}=\overline{\bar A.\bar B}=A.B$$

Which is AND operation